Für die volle Funktionalität dieser Site ist JavaScript notwendig. Hier finden Sie eine Anleitung zum Aktivieren von JavaScript in Ihrem Browser.

zum Inhalt springen
zum Footer springen

Faden-Modelle

Sammlung Mathematischer Modelle am Institut für Geometrie und Topologie des Fachbereichs Mathematik

Schraubenflächen

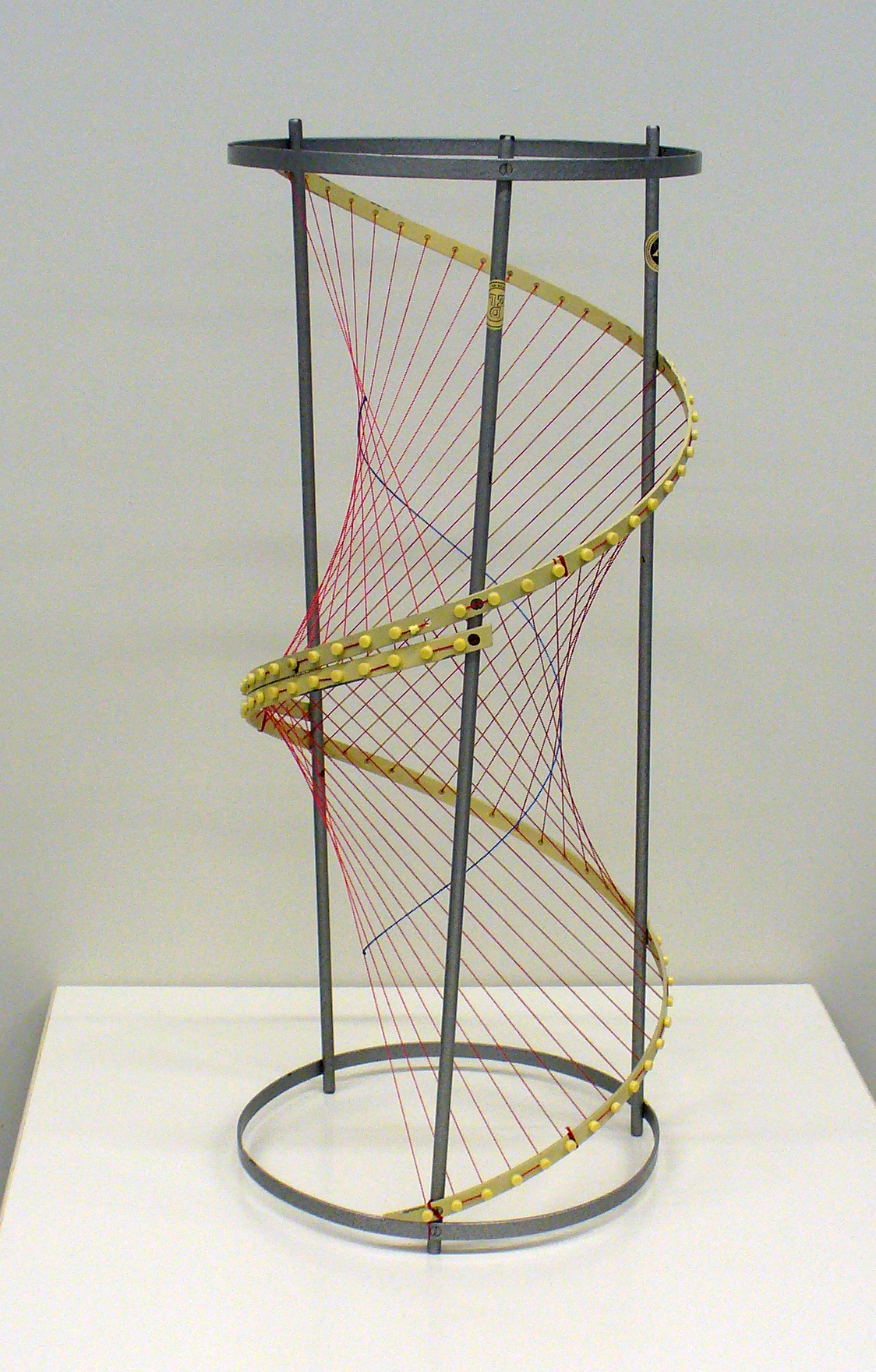
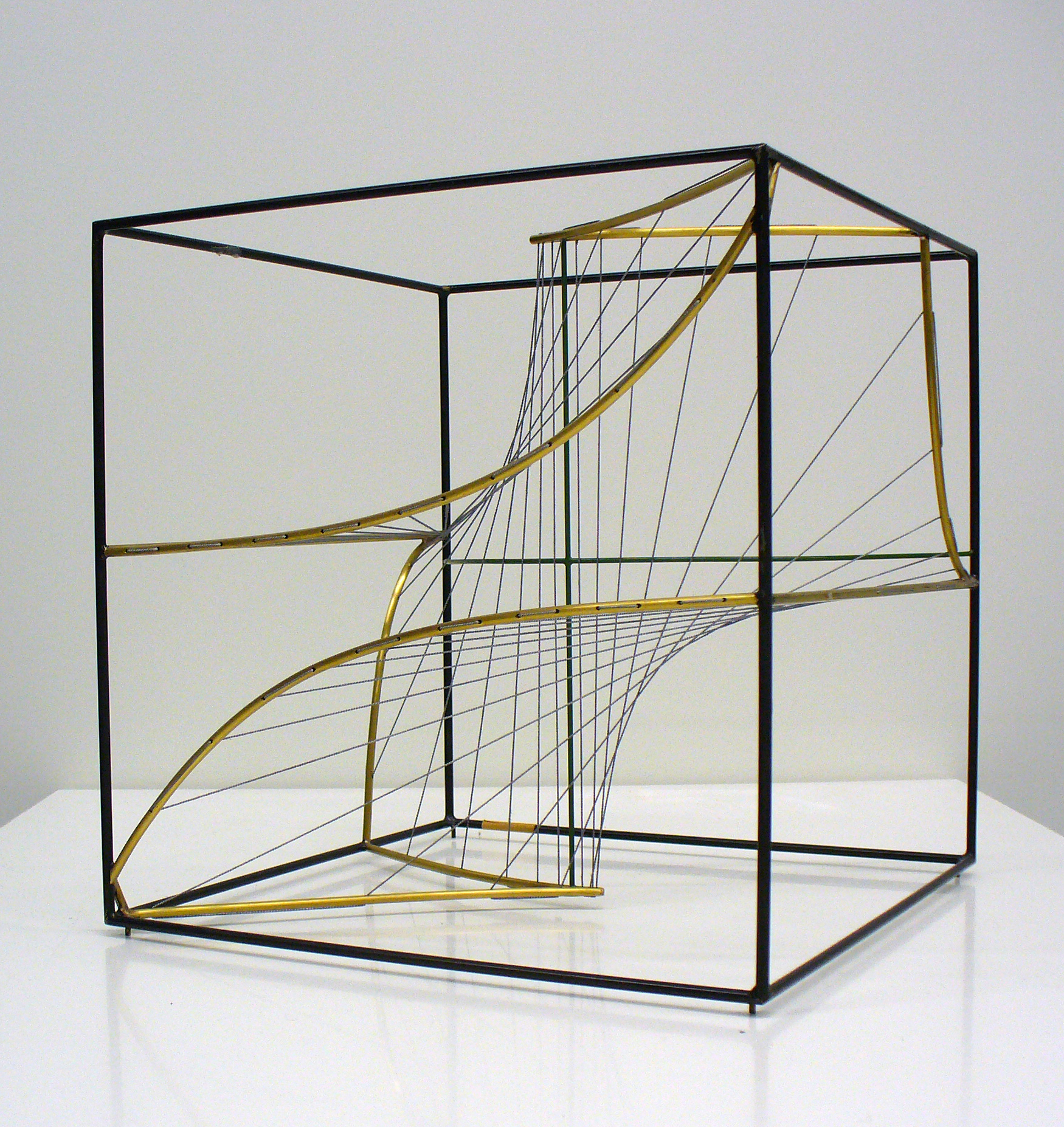
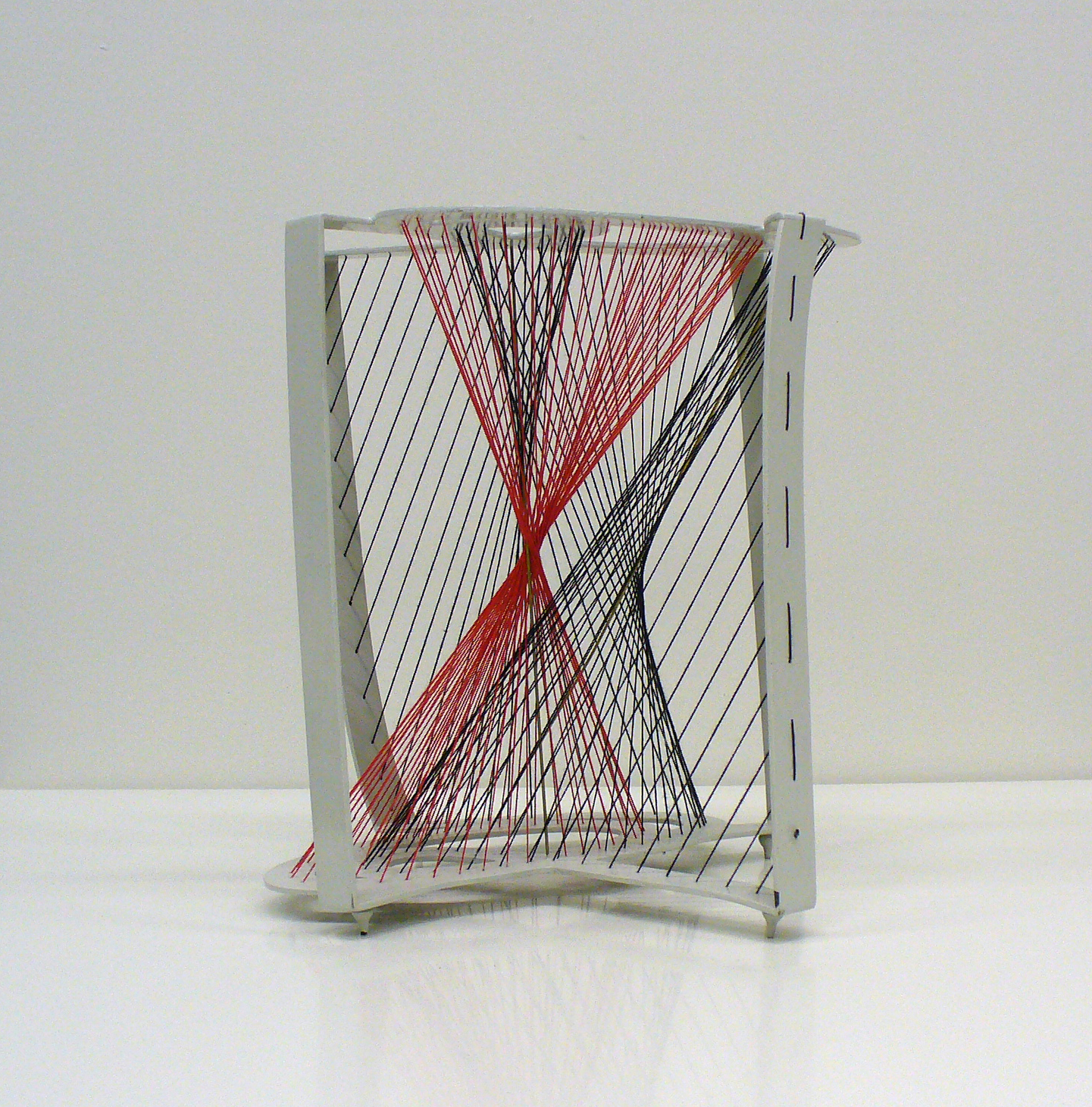
Fm_1 Abwickelbare Regelschraubenfläche (1.Fall) 20 x 21 cm, Metall und Fäden Verlag Martin Schilling Modellnummer: 1a, 127
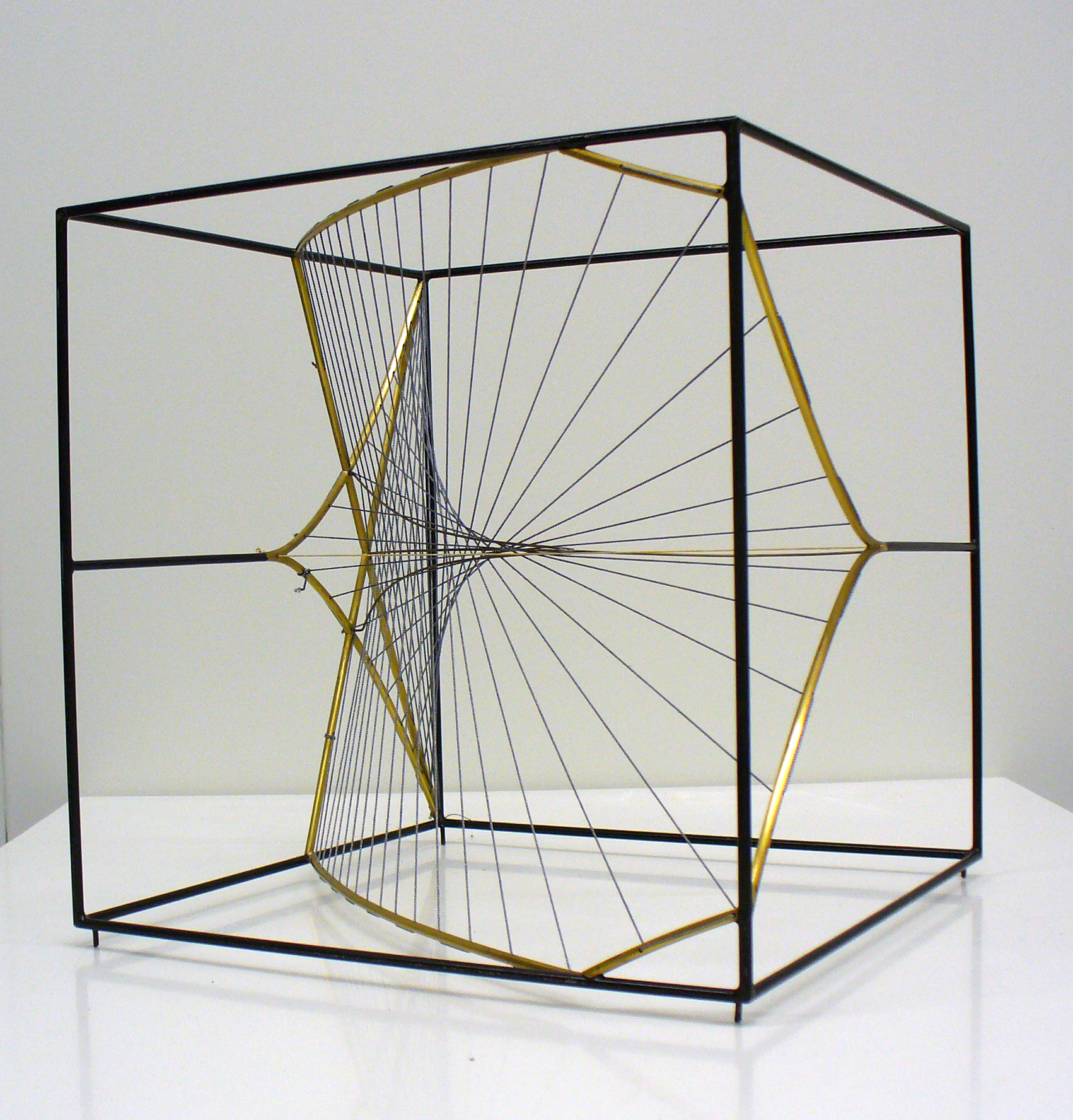
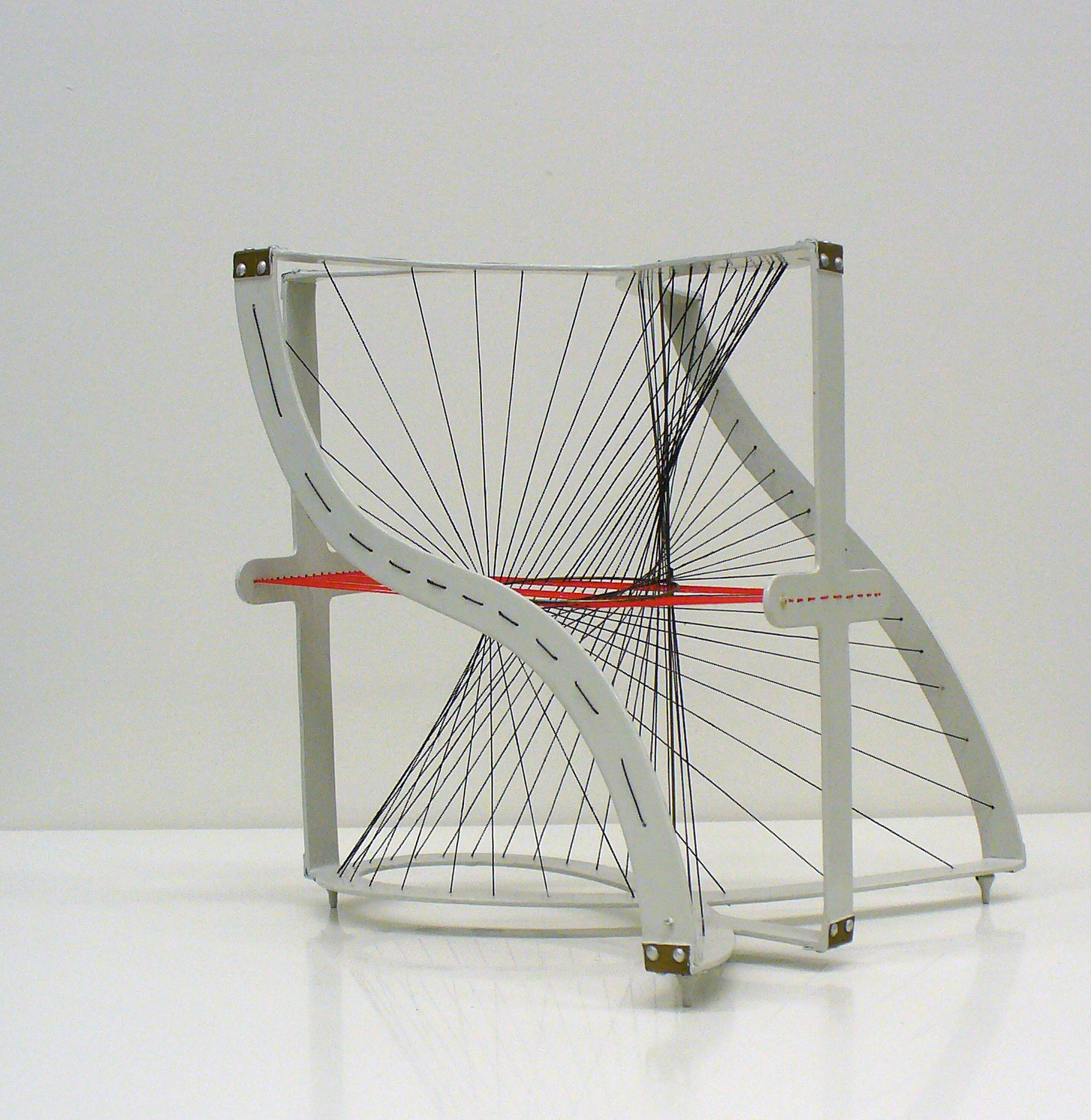
Fm_2 Verschlungene Regelschraubenfläche (2.Fall) Gerade geschlossene Schraubenfläche (Wendelfläche) 20 x 22 cm, Metall und Fäden Verlag Martin Schilling Modellnummer: 2, 129
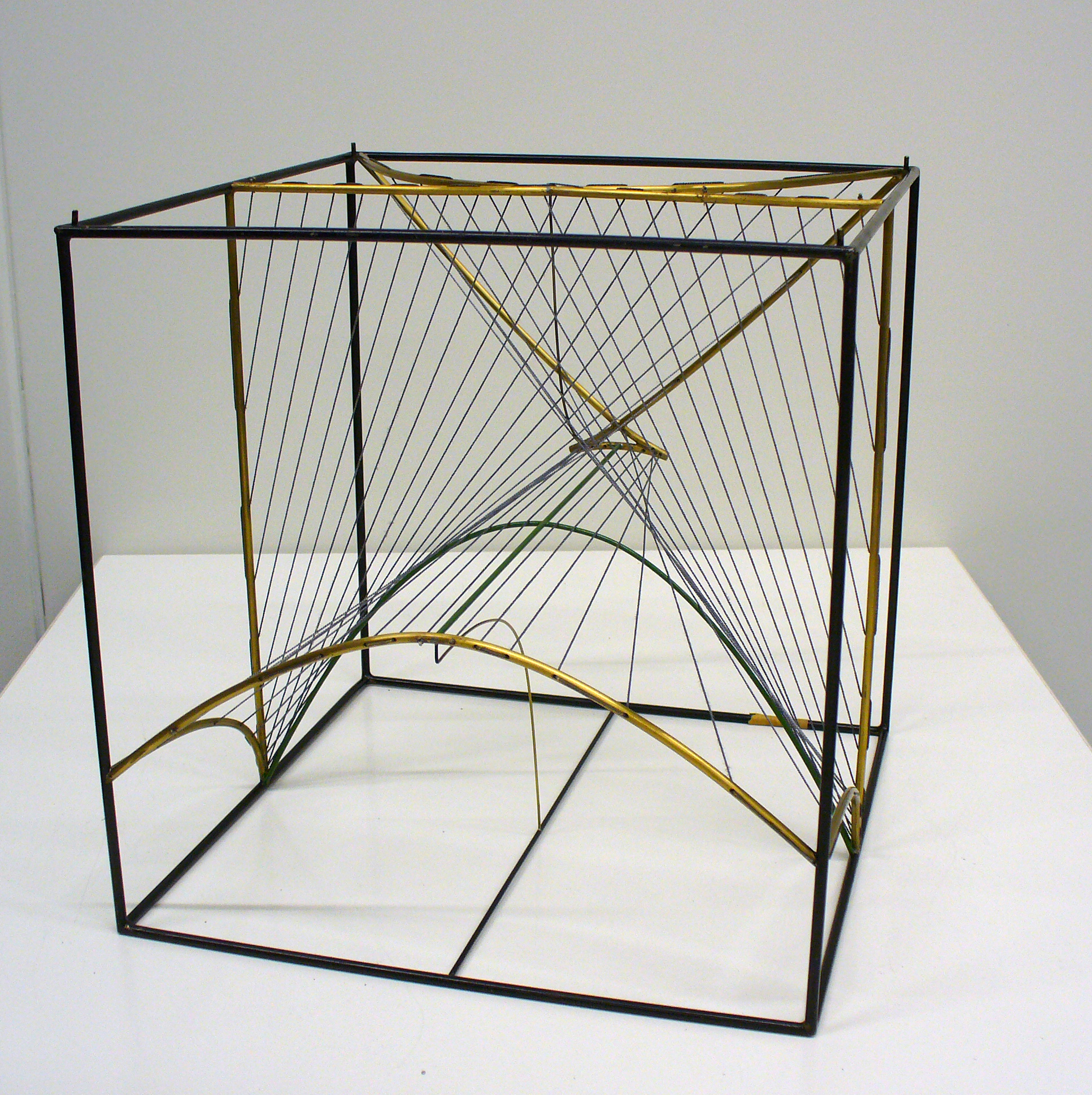
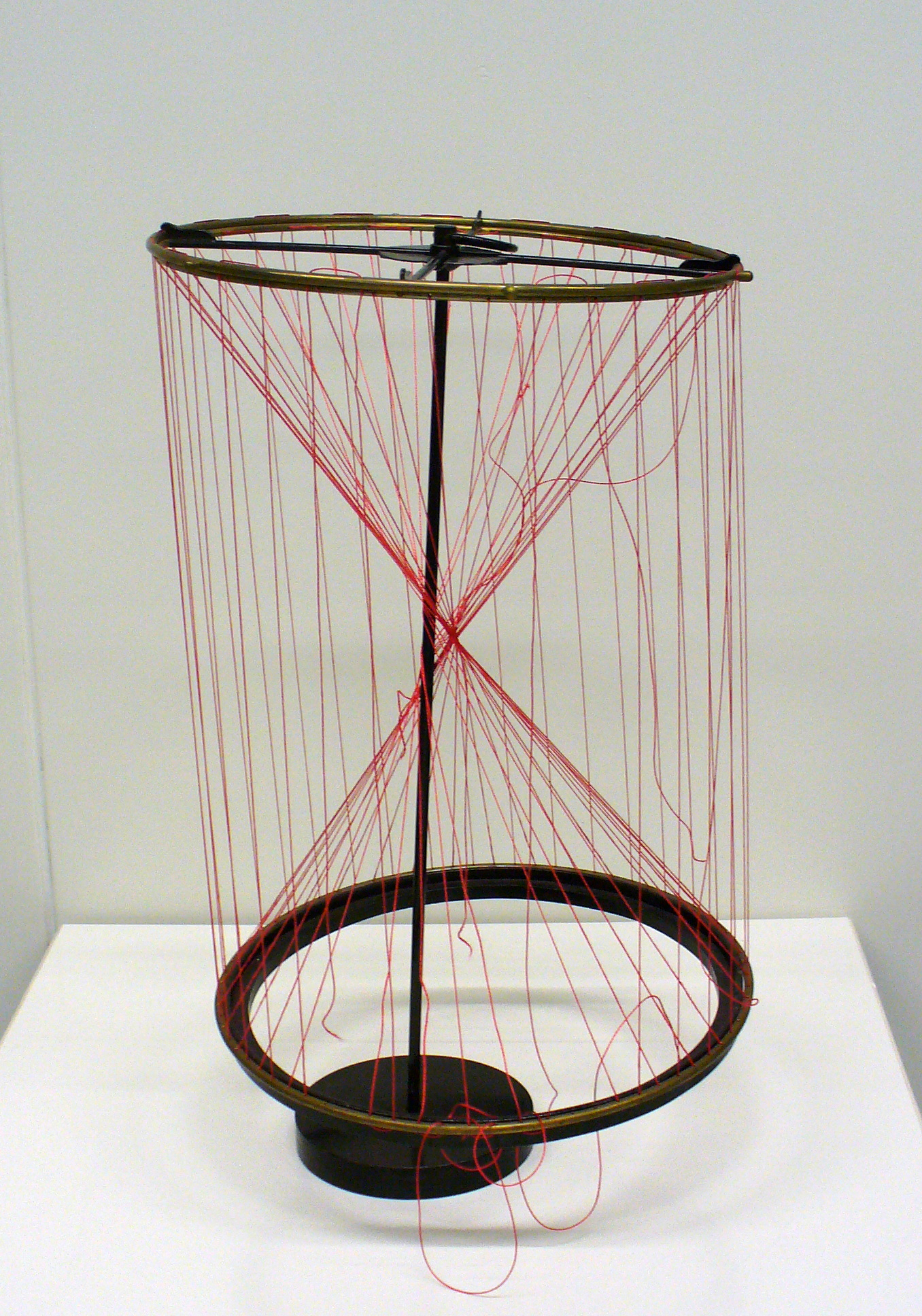
Fm_23 Schiefe offene Strahlschraubfläche 25 x 25 x 57 cm, Metall, Faden
Fm_24 Schraubtorse Tangentialfläche einer Schraublinie (Grad) 25 x 25 x 57 cm, Metall, Faden

Sigularitäten von Raumkurven

Erläuterungen zu Singularitäten von Raumkurven

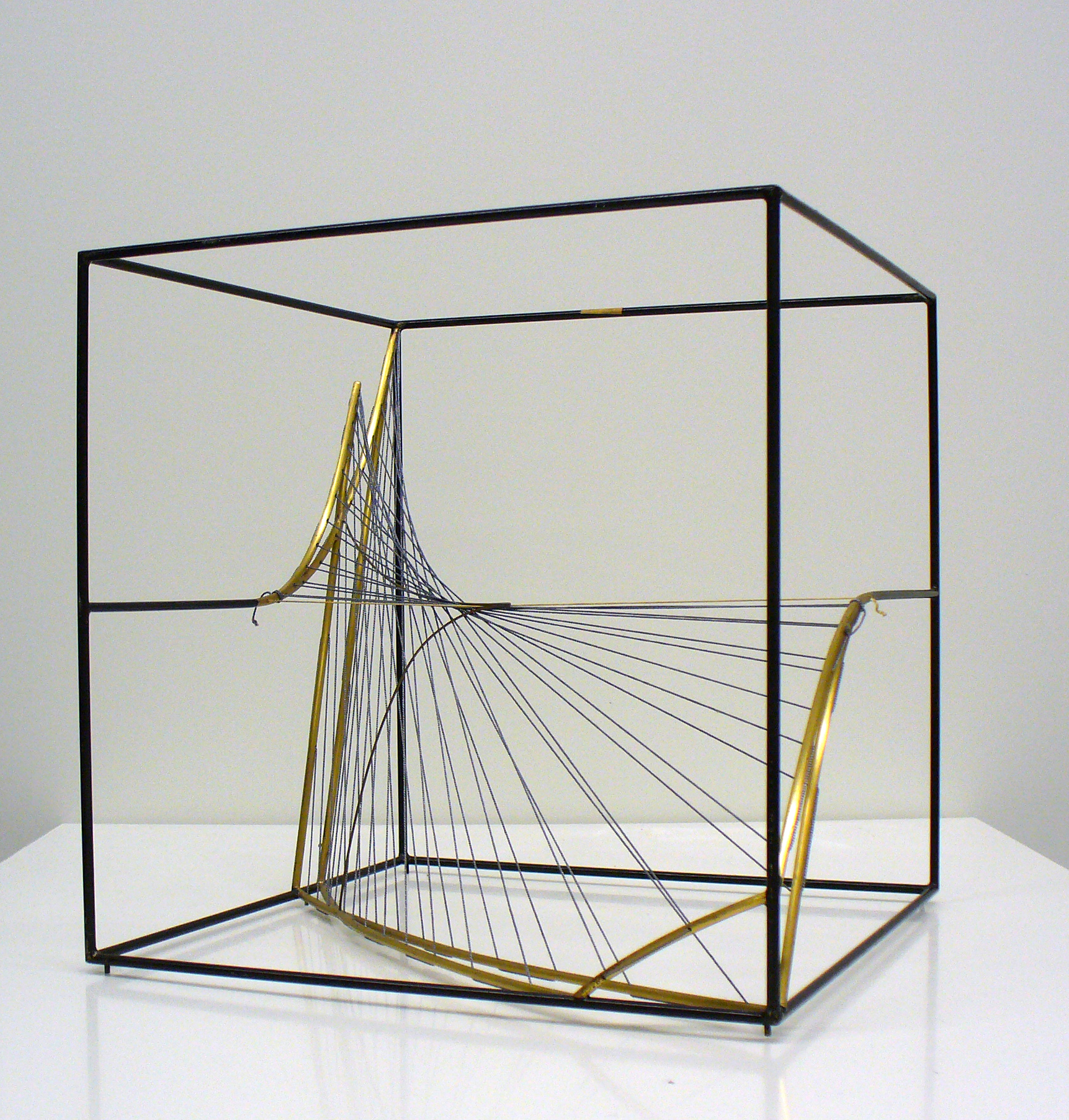
Fm_4 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.26 - Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an improper point and a proper osculation plane as well as a proper osculating plane and an improper point
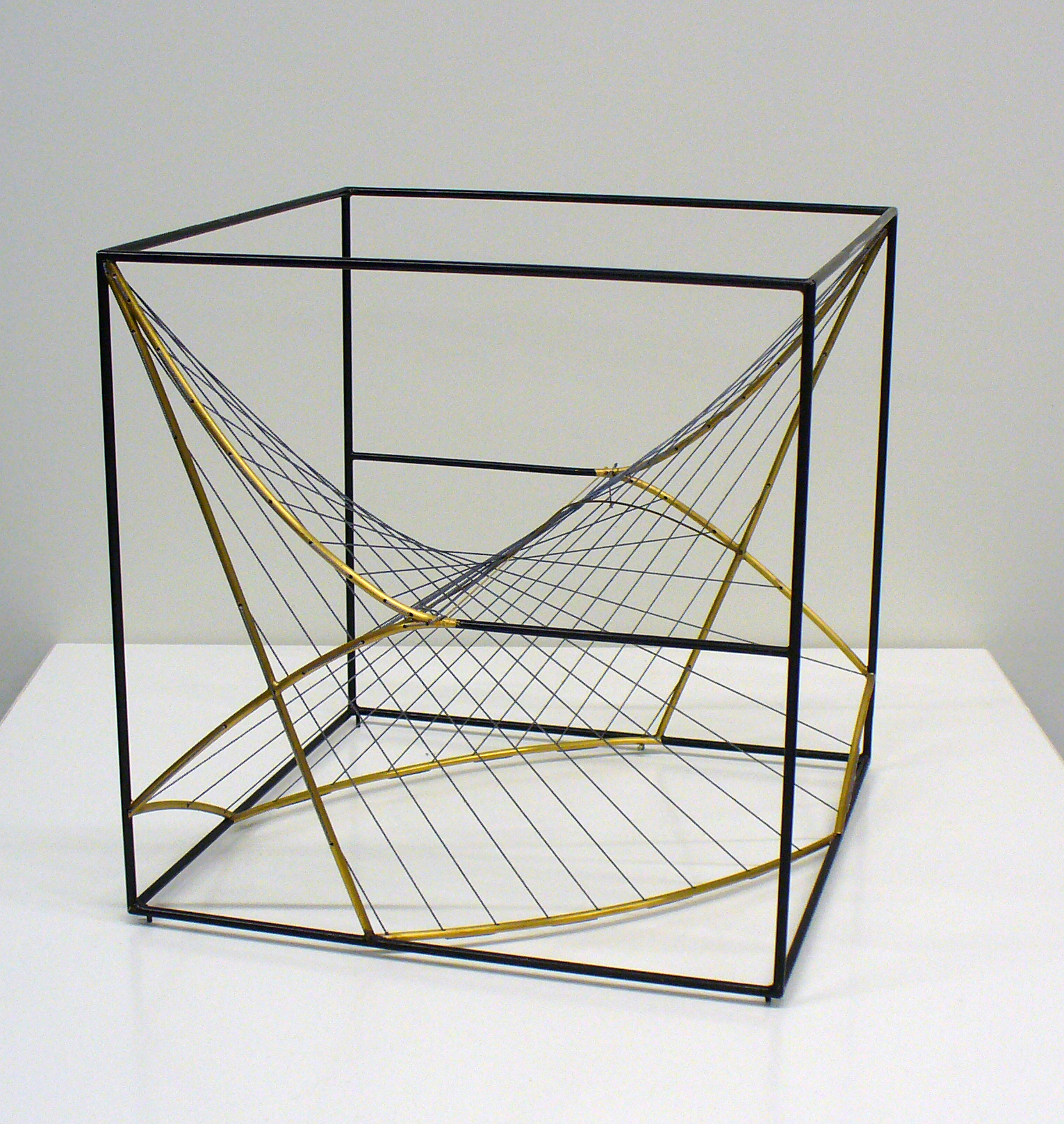
Fm_5 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.11 - Model with uniform positional relation-ships of the curve elements to infinity- Model without reverse elements
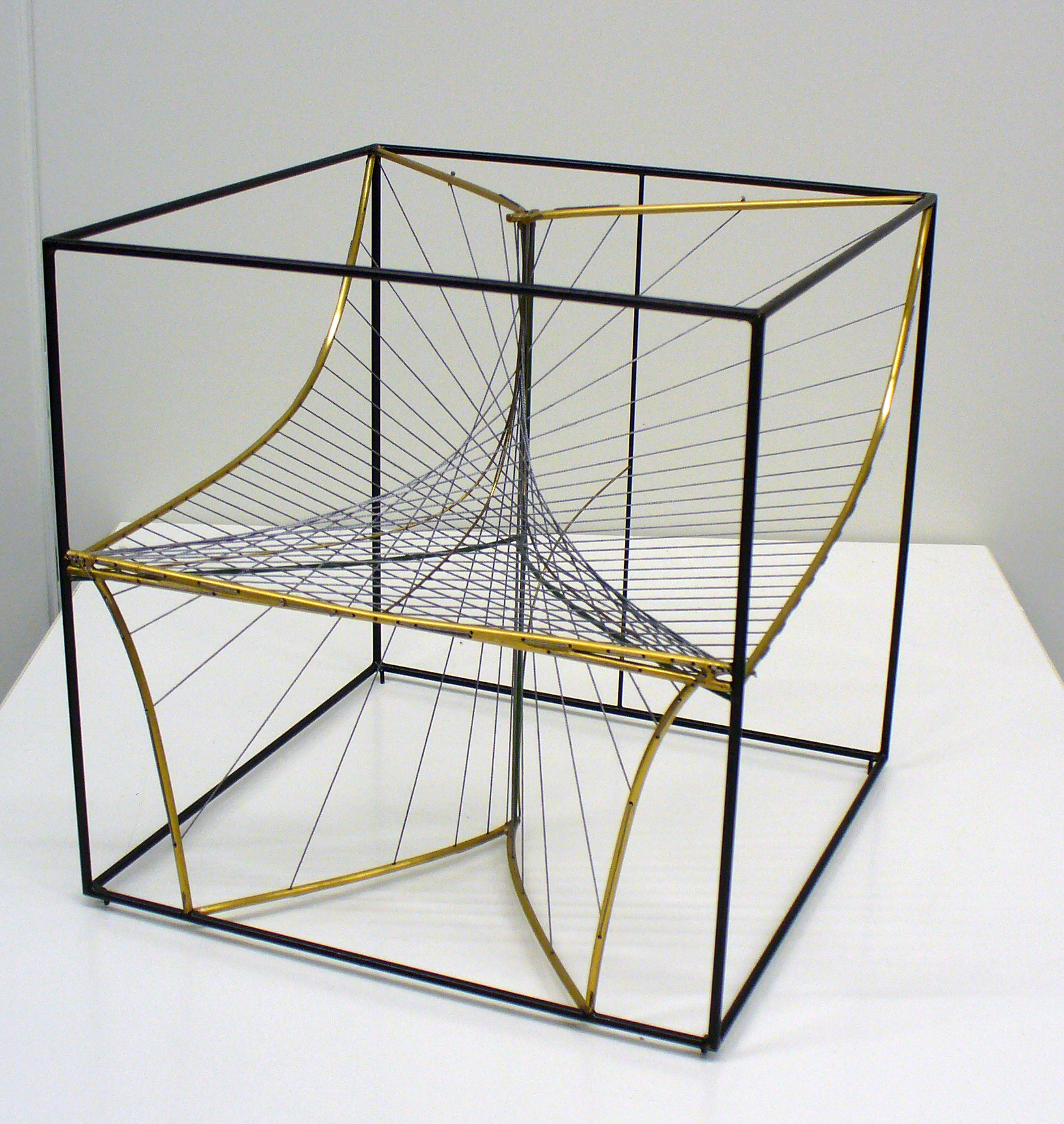
Fm_6 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.16 Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper point, a proper osculating plane as well as improper osculation plane and an improper point
Fm_7 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.28 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper point, a proper tangent and a proper osculation plane as well as an improper oculation plane, an improper tangent and an improper point
Fm_8 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.17 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper tangent and a proper oculation plane as well as an improper tangent and an improper point
Fm_9 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.13 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper tangent as well as improper tangent
Fm_10 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.27, Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper tangent and a proper osculating plane as well as an improper tangent and an improper point
Fm_11 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.25, Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an inproper point and a proper tangent as well as a proper osculating plane and an improper tangent
Fm_12 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.24, Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an inproper osculating plane as well as a proper point
Fm_13 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.14 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper osculating plane as well as an improper point
Fm_14 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. Nr. 16.18, Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an inproper point, a proper tangent and a proper osculating plane as well as a proper osculating plane, an improper tangent and an improper point
Fm_15 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.21 - Model with non-uniform positional relationships of the curve elements to infinity- Modelwithout reverse elements
Fm_16 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.23, Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an inproper tangent as well as a proper tangent
Fm_17 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.15 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper point and a proper tangent as well as an improper osculating plane and an improper tangent
Fm_18 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.22 - Model with non-uniform positional relationships of the curve elements to infinity- Model having as reverse elements an inproper point as well as a proper osculating plane
Fm_19 Singularitäten von Raumkurven 25 x 25 x 25 cm, Stahl lackiert, Messing, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978 Nr. 16.12 - Model with uniform positional relationships of the curve elements to infinity- Model having as reverse elements a proper point as well as an improper osculating plane

Polyeder

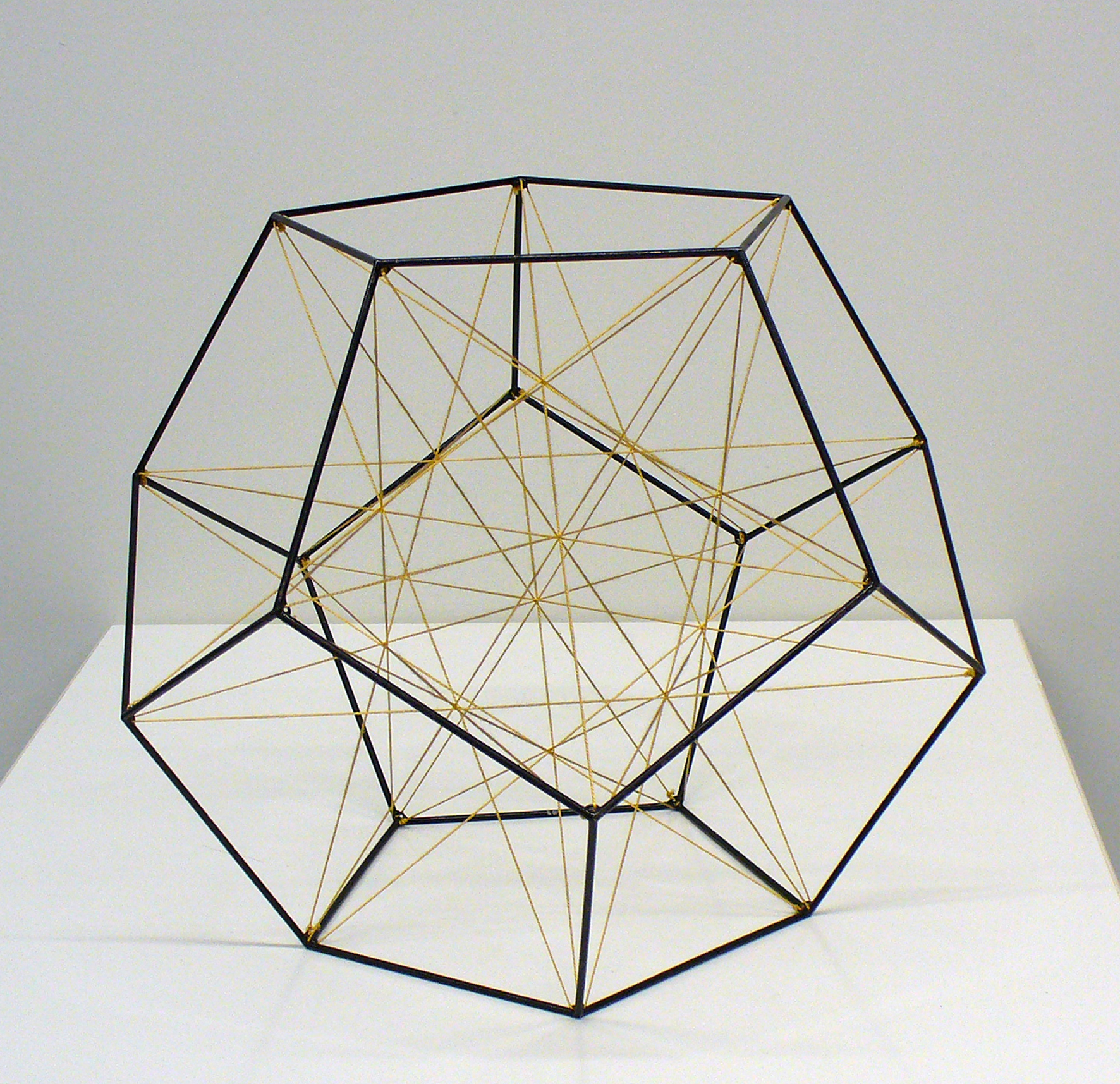
Fm_3 Dodekaeder mit 5 einbeschriebenen Würfeln Kantenlänge 13 cm, Stahl lackiert, Fäden Karl Kolb Frankfurt Mathematische Modelle, 1978
Fm_29 Dodekaeder Kantenlänge 13 cm, Stahl lackiert, Fäden
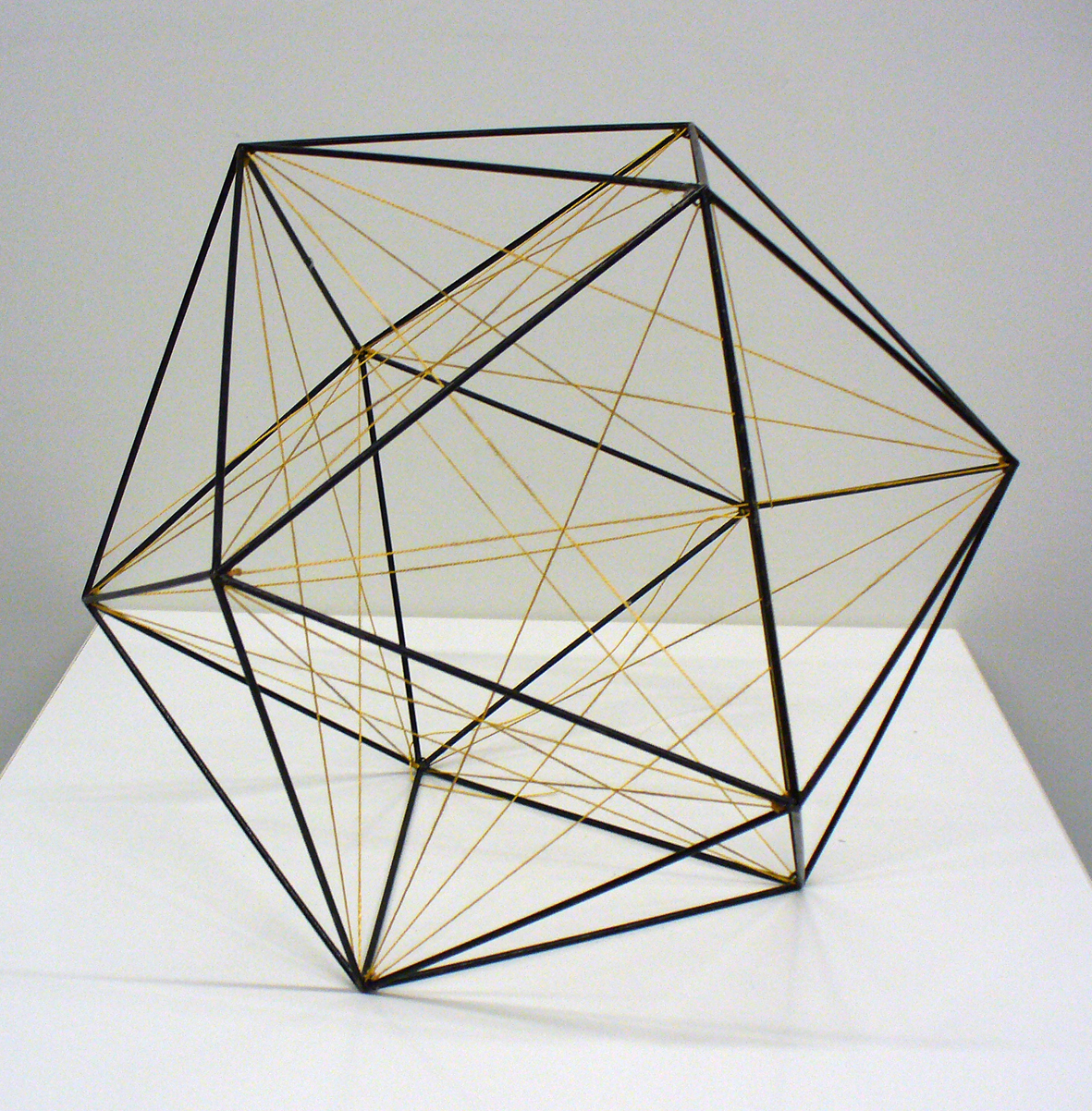
Fm_30 Ikosaeder Kantenlänge 20 cm, Stahl lackiert, Fäden
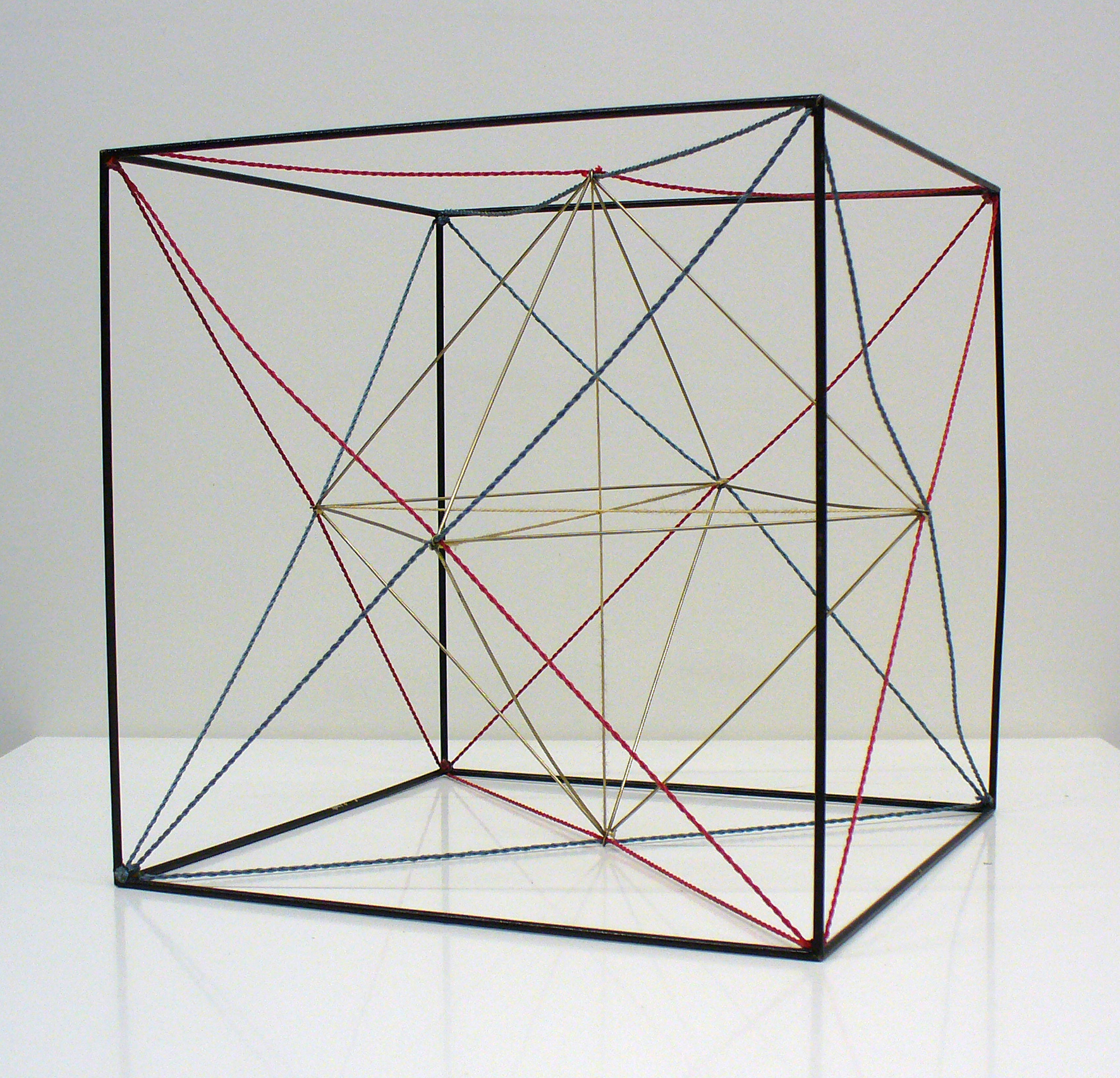
Fm_31 Hexaeder Kantenlänge 25 cm, Stahl lackiert, Fäden

Regelflächen 3. Ordnung

Erläuterungen zu Strahlregelflächen 3. Grades

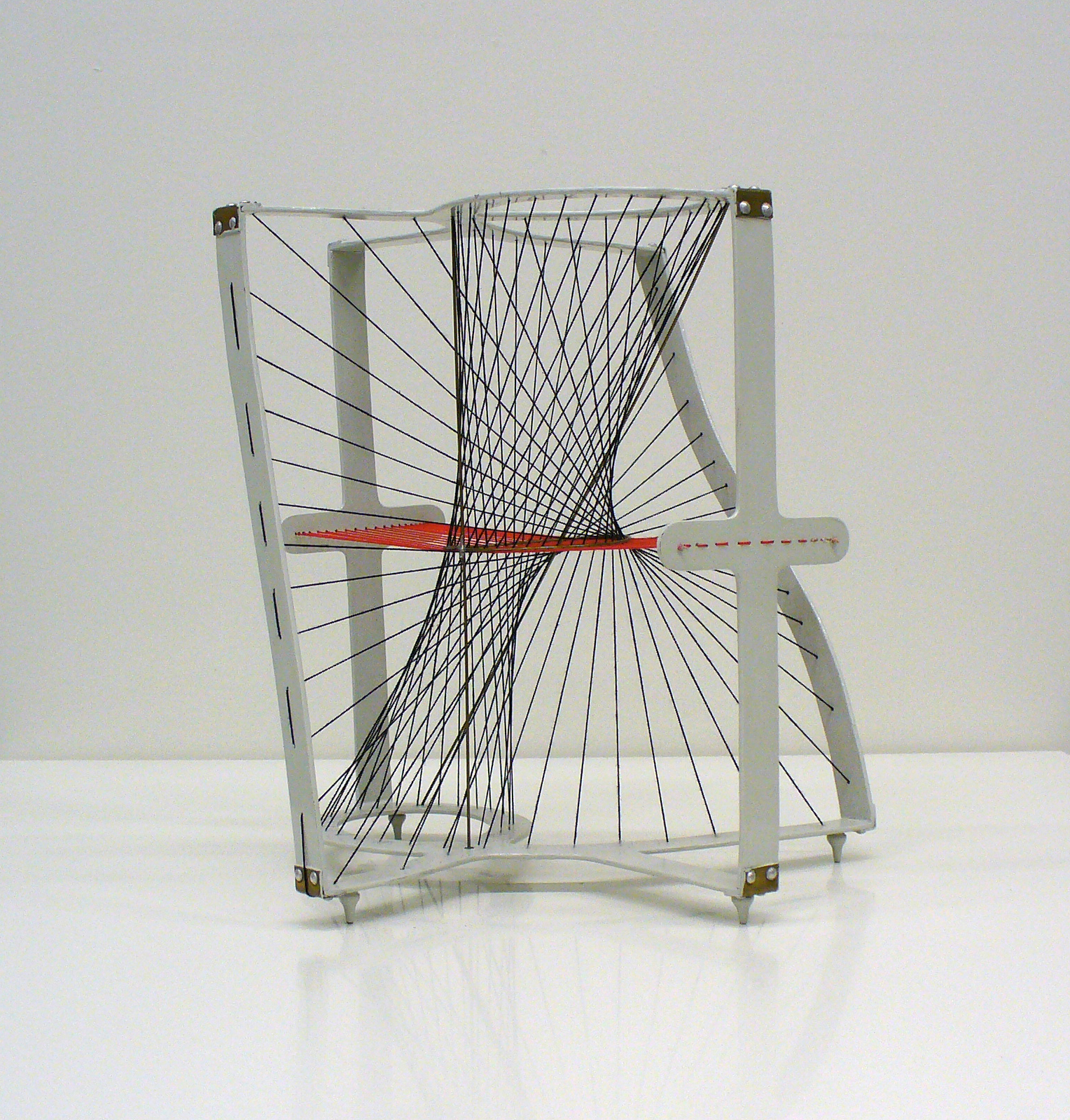
Fm_27 Regelflächen 3. Ordnung 1. Fall Die 2. Leitgerade schneidet die Ebene des Kreises in einem innerhalb desselben gelegenen Punkte. Die 1. Leitgerade ist ihrem ganzen Verlauf nach reelle Doppellinie der Fläche. Die Kanten und Cuspidalpunkte sind imaginär. Zitiert nach Schilling, M.: Catalog mathematischer Modelle für den höheren mathematischen Unterricht, 6. Auflage (1903), S. 88. 20 x 20 x 20 cm Metall, Faden
Fm_25 Regelflächen 3. Ordnung 2. Fall Die 2. Leitgerade schneidet die Ebene des Kreises in einem außerhalb desselben gelegenen Punkt. Die 1. Leitgerade ist längs einer endlichen Strecke reelle Doppellinie in den beiden sich in das Unendliche erstreckenden Zweigen isolierte Linie der Fläche. Es sind 2 reelle Kanten vorhanden, welche durch die beiden Grenzpunkte des reellen Teils der Doppellinie gehen. Diese Grenzpunkte sind Cuspidalpunkte der Fläche. Zitiert nach Schilling, M.: Catalog mathematischer Modelle für den höheren mathematischen Unterricht, 6. Auflage (1903), S. 88. 25 x 25 x 20 cm Metall, Faden
Fm_26 Regelflächen 3. Ordnung 3. Fall Der Leitkegelschnitt, hier eine Ellipse, ist in das Unendliche gerückt und durch einen Richtkegel gegeben, der durch seine Erzeugenden dargestellt ist. Die 2. Leitgerade schneidet die Ebene des unendlich fernen Kegelschnittes in einem außerhalb desselben gelegenen Punkt. Ein endlicher Teil der ersten Leitgeraden ist isolierte Linie, die beiden sich ins Unendliche erstreckenden Zweige reelle Doppellinie der Fläche. Die Kanten und Cuspidalpunkte sind wie im 2. Fall reell. Zitiert nach Schilling, M.: Catalog mathematischer Modelle für den höheren mathematischen Unterricht,6. Auflage (1903), S. 88 21 x 21 x 20 cm, Metall, Faden
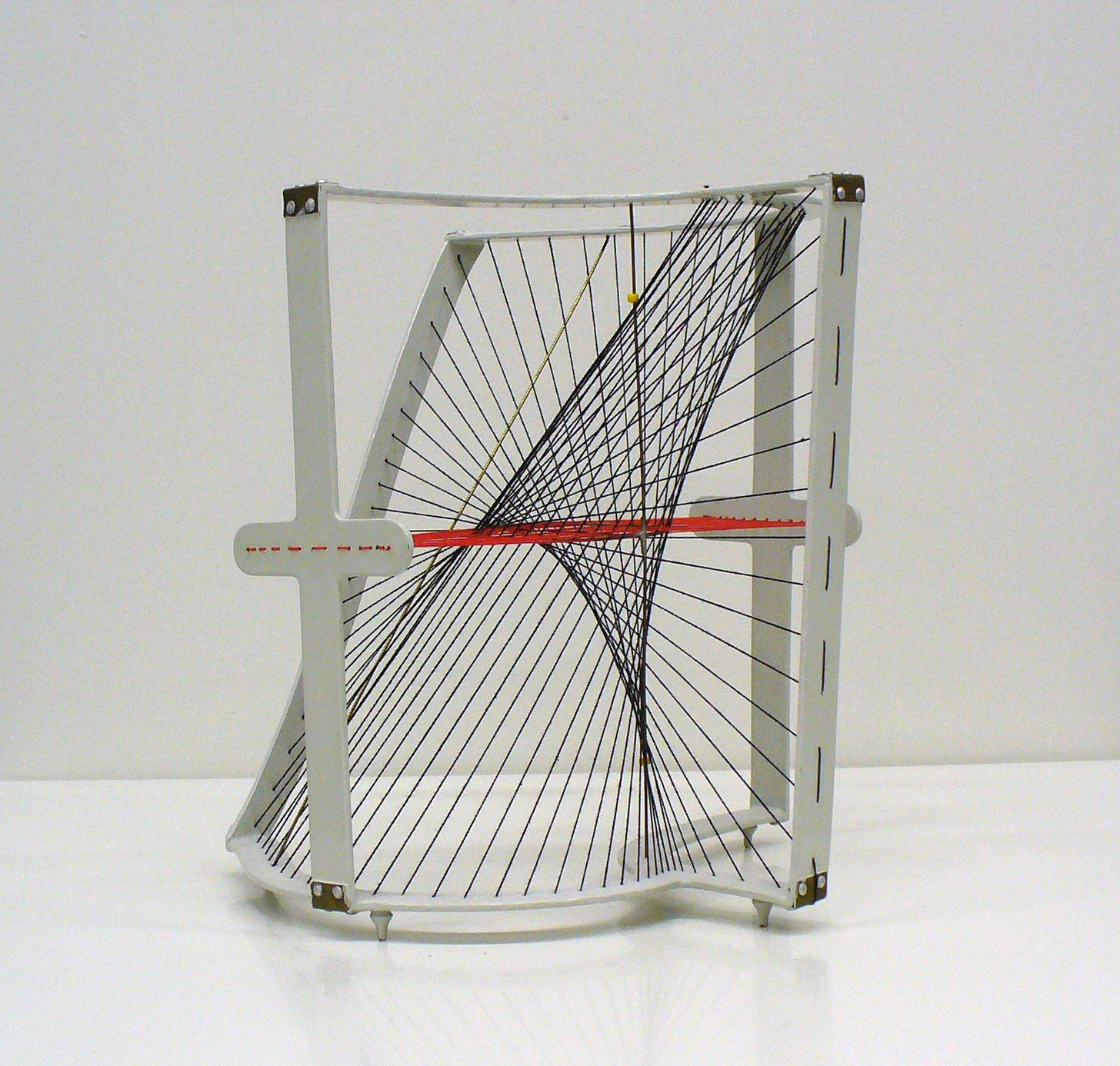
Fm_28 Regelflächen 3. Ordnung 4. Fall (Cayley'sche Fläche) Die 2. Leitgerade fällt mit der 1. zusammen. Dieselbe ist in ihrem ganzen Verlauf reelle Doppellinie und zugleich Kante der Fläche. Die beiden Cuspidalpunkte fallen in einem Punkt zusammen, durch welchen Punkt noch die 2. Kante der Fläche geht. Zitiert nach Schilling, M.: Catalog mathematischer Modelle für den höheren mathematischen Unterricht,6. Auflage (1903), S. 88 21 x 21 x 20 cm Metall, Faden

Weitere Fadenmodelle

Fm_32 Zylindroid, vereint mit dem rechtwinkligen Paraboloid 8 x 15 cm, Metall, Faden
Fm_20 Einschaliges Hyperboloid mit Asymptotenkegel 23 x 23 x 23 cm, Kunststoff, Faden zweifarbig
Fm_21 Einschaliges elliptisches Hyperboloid mit Asymptotenkegel 23 x 20 x 23 cm, Kunststoff, Faden zweifarbig
Fm_22 Drehhyperboloid 30 x 30 x 45 cm, Metall, Faden

Zurück zur Übersicht der Sammlung Mathematischer Modelle

Zum Seitenanfang